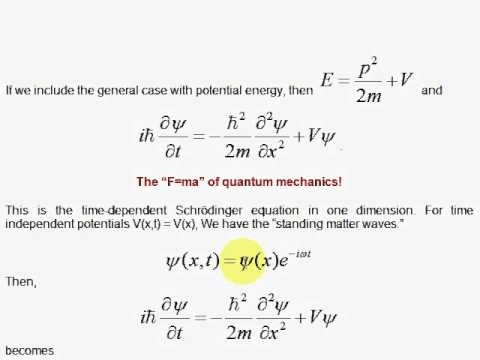
This restriction might seem as overly restrictive to the class of solutions of the Schroedinger Equations, but in this case appearances are deceiving. One of the functions is a function of the position alone whereas the other function is solely a function of. We’ll assume that ca be written as the product of two functions.
To do such a thing we’ll employ the separation of variables technique. The trick is to to turn this partial differential equation into ordinary differential equation. Partial differential equations are very hard to solve whereas ordinary differential equations are easily solved. Which is partial differential equation of second order. You have to solve the Schroedinger equation. The answer to that question obviously is: How does one calculate the wave function in the first place? In the previous posts we’ve normalized wave functions, we’ve calculated expectation values of momenta and positions but never at any point we’ve made a quite logical question: